Become a Member
Become a Member
LIBRARY
  2. Library
  3. Viewnugget
  4. 487

Steady-State Analysis of Voltages and Currents in the Modular Multilevel Converter Based on an Average Model

Year: 2015
Fig. 1. (a) Three-phase MMC inverter and (b) Half-bridge submodule as building-block of MMC AFE
The modular multilevel converter (MMC) is being considered as the next generation converter among multilevel topologies, and with the introduction of MMC, a new era has opened in the field of medium, and high-voltage, high-power converters. Sizing the passive elements and design of the converter along with its performance evaluation is thus of great importance for researchers in this area. This paper provides a steady-state analysis of the modular multilevel converter (MMC) based on an average model. The magnitudes and phase angles of current and voltage quantities are calculated herein. Further, equations are solved for different components of the circulating current and submodule voltage, and the resonance behavior in circulating current harmonics is investigated. Based on the resonance behavior of circulating current harmonics, a guideline is given for choosing the magnitude of submodule capacitance and arm inductance. A model is developed in the MATLAB/Simulink environment in order to verify the performed calculations' accuracy.

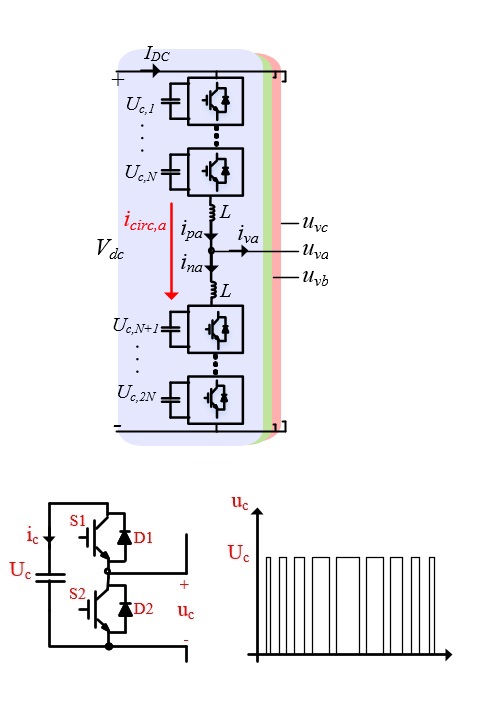
The wide variety of applications, along with the need for a significant amount of passive elements installed, requires the best possible MMC converter design. In order to understand the operation of the MMC and then design the converter for the target application, an accurate analysis of the voltage and current waveforms in the MMC are of great importance.

In this paper, a detailed analysis of submodule voltages and arm currents is done based on an average model. The analysis takes into consideration the arm current components up to the 4th, and submodule voltage ripple components up to the 5th, harmonic. Also, the resonant behavior of the 2nd and 4th order harmonics in the circulating current is investigated and the relationship between resonance frequency and the magnitude of passive elements is derived. Then, a guideline to select the size of arm inductance and submodule capacitance is discussed. Finally, validity of the derived equations is shown via simulations in MATLAB/Simulink.

Our Industry Partners

About CPES

Events/Presentations

Education

Interested in Becoming a CPES Member?

Learn more


Having technical issues?

Get Help

Copyright © 2021 Virginia Tech Center for Power Electronics Systems