LIBRARY
Stability Analysis of Power Systems With Multiple STATCOMs in Close Proximity
Year: 2020 | Author: Ye Tang | Paper: T4.16
Fig. 1. Potential instability due to ac voltage loop.
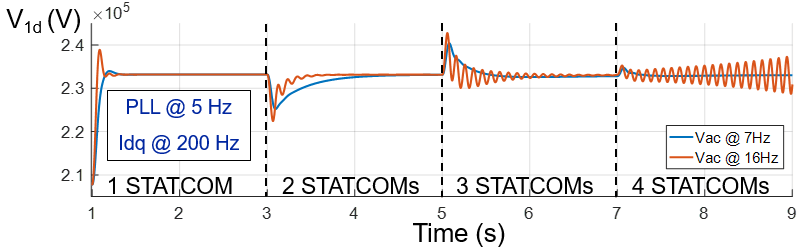
In this work, potential instability in power systems with multiple STATCOMs in proximity was found due to the design of STATCOM controllers: the ac voltage controller, the PLL, and the QV droop controller. A dq frame impedance-based stability analysis was used to explore the instability. From the analysis, the following conclusions were obtained. First, STATCOMs were seen to interact with each other through the transmission lines due to the relatively high magnitude of their impedances with respect to those of the lines. Second, the use of dq frame impedances identified the frequency range of interactions. The ac voltage regulation was the main reason for instability among STATCOM controllers and masked the effects of PLL in transmission systems. This kind of instability adds new knowledge about three-phase ac systems, besides CPL dynamics and synchronization, with the presence of STATCOMs. Although STATCOMs synchronize with the power grid, the instability is nonetheless due to ac voltage regulation. Third, due to the large impedance of STATCOMs around the frequency range of interactions, the number of connected STATCOMs was the main contributor to stability instead of the topology of the power system or the locations of the STATCOMs. The high magnitude of STATCOM impedance around oscillatory frequency range makes it the dominant reason for instability, while the topology of the power systems showed negligible influences. The use of alternative controllers could improve stability conditions, as they can damp the resonant peak in the STATCOM impedance, which could cause instability. However, this cannot avoid the onset of instability. Finally, a scaled-down, two-STATCOM power grid was built to verify the conclusions experimentally. Finding parameters that help in stabilization using the impedance-based method is essential but not easy because it is hard to correlate a specific control parameter to stable regions using simplified and straightforward relationships, as the exact parameter ranges that stabilize the system vary with the system parameters, such as line impedances, load flows, etc. This will be a future path of exploration.
